Breaking Bad...Definitions

I posted a picture on Twitter the other day (see below) that included the 8 SMPs in "Kid Speak".  Ashli Black (@Mythagon) pushed back on #6, saying that precision is more than just numerical accuracy, but also precision of language.  I could not agree more!

I have found that necessitating the precision of language is fairly easy in my classroom because my students do a ton of talking and writing.  But I think the major difference is that they share their writing and verbal thoughts with their partners and the whole class, so I'm not the only audience member, which seems to be more important to the kids.  (Remember, these are teenagers.  They care very much about what their peers think.)

We were getting into our unit on polygons and I wanted to try out an activity that my colleague Dr. Patrick Callahan had shown me a few years ago.  I was actually attending a PD session at UC Davis where he was presenting and he asked all of us (a room full of math educators) to write down our definition of the word "polygon".  I've adapted the activity slightly and I used it with my students just the other day.

We started with this handout:

The instructions read:

"The purpose of this activity is to work toward a mathematically viable definition of the term “polygon”.  To begin, write a first draft of your definition.  Then, exchange papers with a peer.  When you read your peer’s definition, consider if you can “break” it.  To break a definition, draw a picture that does satisfy their definition, but is not a polygon.  We will have a mathematically precise definition of polygon when we cannot draw a picture to break that definition.

            For example, if we were trying to define the term “vegetable”, my first draft might be: A vegetable is a food.  When I exchange papers with my partner, they might break my definition by writing the word “chicken”.  This breaks my definition because it does satisfy my proposed definition, but it is not a vegetable."

I should say that I was essentially looking for the following elements in an "unbreakable" definition of polygon:

• Closed
• 2-Dimensional
• Straight sides
• At least 3 sides/More than 2 sides
• Sides that intersect only at endpoints

I asked the students to begin writing down their initial ideas of what a polygon is.  Many got right to it, others stalled to wait and see what others wrote, and some got flustered, panicked, and asked me "What if I have no idea?"  I reassured them that we were not going for the right answer at this stage and I just wanted them to put down ANYTHING that they knew about the word.  This seemed to help a bit.

I walked around and saw a variety of first drafts:

polygon - a shape

A polygon is a shape or a figure

A closed shape with more than 2 sides

A polygon is a closed shape with no curved edges.

A shape with more than one side

A shape with 6 sides

 As papers started to move around for definitions to be broken, I found that this part was really challenging for some students.  "How am I supposed to break their definition if I don't even know what a polygon is?"

I grabbed a sample paper (see below) and put it under the document camera.  The first draft read: "polygon - a shape".  I asked the students how we might break this definition of polygon and one student said, "You could draw a circle.  Because even though it is a shape, it is not a polygon."  This started a lot of conversation at each table and students were brainstorming ways that they could break the definition on the paper in front of them.

(as mentioned above)

Here are some ways that students broke the first drafts:

This student was calling out the fact that sides could not be curved

This student was addressing the fact that a polygon could actually have less than 5 sides

Now, not all students really got the way that the breaking process was working.  Here are some examples of pictures that did not actually break the proposed definition:

"A polygon is a shape that has multiple sides and is not round" is not actually broken by a circle

"A polygon is a geometric figure".  And so is the shape drawn in the box.

It was at this stage that I realized that my description of "breaking" a definition was not precise enough.  In my vegetable example, I had gone in the direction of not being specific enough in my definition, or as Dr. Callahan would say, I've "included too much".  I hadn't considered the other direction of not including enough.  In the example above where the student broke the definition by drawing a trapezoid, the original definition had left out figures with 3 and 4 sides, which had excluded too much.  This was an opportunity to revise my own definition of "breaking".  Obviously I always love a great opportunity to model what I am asking my students to do!

Also at this point, students got their papers back and started revising.  You can see that the precision of language was called out without me really having to say too much.  There was a sense of competition that students had with one another because they wanted to come up with an "unbreakable" definition before anyone else did.  Take a look at their second drafts:

(Please note that not all of these are great, but you can see that students were attending to the precision of their language, regardless of whether or not the picture actually broke their previous draft.  Many students relied on the examples show to the whole class under the document camera or other papers that they saw to revise their definitions.)

From this point on, I didn't need to do a lot of modeling to the whole class, they were switching papers back and forth on their own.  Students would call me over if they thought they had an unbreakable definition and I might tell them that there was still a way to break it, or I might pose it to the whole class to decide.  Could they break it?

Here are a couple examples of further revisions:

Also, after a few rounds of revision, most students were good with their definitions including closed figure, straight sides, and at least 3 sides.  Now, it was time for me to push them on the piece that could describe the requirement of sides only intersecting at endpoints.

I drew these images on the board:

They knew that the first picture was unacceptable because it wasn't closed.  They were fine with the middle image.  But I told them that I could break a lot of their existing definitions with the third picture.

A lot of students said, "Sides can't intersect!!!" would be their revision.  This is when I pushed back with, "Well, you can't have both.  You can't say that it's a closed figure AND that sides don't intersect."

Silence.

I asked them to brainstorm with their group how they might describe the difference between the middle picture and the one on the right.  What made one acceptable, but not the other?  Check out what they came up with:

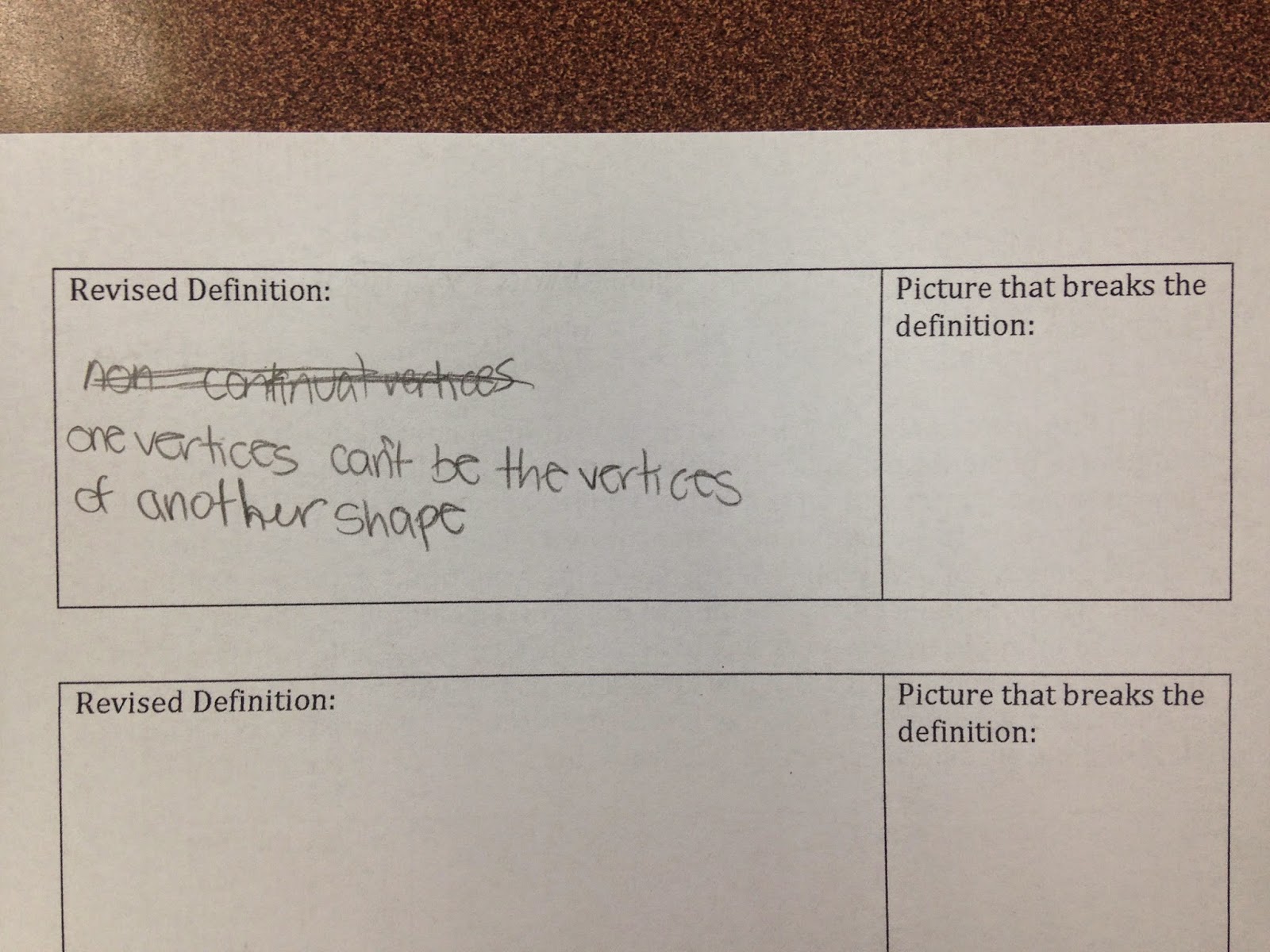
She told me later that she meant "vertex" not "vertices"

This student actually came up with this language before I addressed it with the class

This is one of my top performing students.  He had most of it down from the beginning.

This last definition is one that the group came up with together

You can see this student's process and her final product below

See above

I loved this idea of the vertical angles connection

At the end of the day, of course, I was wanting my students to have a solid understanding of what the term "polygon" means, but this activity allowed for so much more than that.  I know that I mentioned the importance of the precision of language, but another layer of this is the process of determining if something "belongs" or not.  Furthermore, if I think it should belong, but my definition doesn't allow for it, I need to revise my definition.  Or, if I don't think it belongs and my definition does allow for it, again, revision is needed.  Dr. Callahan really made this clear for me when I debriefed this activity with him.  It was something that I had not really considered, but was so powerful for students.

And just to wrap it all up, I had to share Dr. Callahan's creative side...

You're welcome.